CONTOUR POLYGONAL APPROXIMATION USING THE SHORTEST PATH IN NETWORKS

Andre Ricardo Backes and Dalcimar Casanova and Odemir Martinez Bruno

INTERNATIONAL JOURNAL OF MODERN PHYSICS C, 25:, 2014

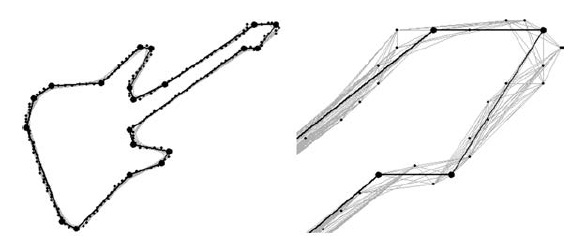
Contour polygonal approximation is a simplified representation of a contour by line segments so that the main characteristics of the contour remain in a small number of line segments. This paper presents a novel method for polygonal approximation based on the Complex Networks theory. We convert each point of the contour into a vertex so that a regular network can be modeled. Then we transform this network into a Small-World Complex Network by applying some transformations over its edges. We compute the polygonal approximation analyzing the network properties, especially the geodesic path. The paper presents the main characteristics of the method, as well as its functionality. We evaluate the proposed method using benchmark contours and compare its results with other polygonal approximation methods.